Introducing Takis Konstantopoulos, one of our Contributing Editors.
Takis did his basic studies at NTUA in Greece where he learned a lot about many subjects (“from complex analysis to quantum mechanics, signal processing, control…”) and his MSc (on Lévy’s Brownian motion) and PhD (in stochastic networks) at UC Berkeley. He says, “I have a tremendous curiosity about everything and enjoy connections. This is probably what made me an academic random walker from INRIA in France, UT Austin, Heriot-Watt in Edinburgh, to Uppsala in Sweden as a chair in Mathematical Statistics, always leaving a tenured position to go to the next one. I love honest applications as much as I love theory.”
A student walked into my office to say hi, a couple of years after graduating with an MSc degree, and asked, “Why didn’t you teach us the central limit theorem for dependent random variables, e.g., martingales? These are the kind of things we need at work.” (He was working in the late 90’s for an internet company on the East coast.) I replied that he was right but there’s a limit to what can be covered in a one-semester course of graduate-level probability for a mixed student audience. I was, however, very pleased to realize, once again, that it is often applications that demand more theory.
I would like to talk here about stochastics (in its all-encompassing sense), mathematics and applications, and about teaching and learning.
I do not understand the distinction between teaching and research, or the difference between theory and applications. How can one teach without being curious to discover something? What’s wrong with having an honest applied viewpoint but also being interested in theory per se? David Blackwell once said, “Basically, I’m not interested in doing research and I never have been. I’m interested in understanding, which is quite a different thing. And often to understand something you have to work it out yourself because no one else has done it.” This is a great attitude. If you have this attitude then, undoubtedly you also think about how to explain what you do. Unfortunately, we are told that differences ought to exist, but who says so, and for what purpose?
One problem with stochastics is that undergraduate education in “natural sciences and engineering” follows by and large the Newtonian tradition. Sets are things that a student visualizes, functions are smooth, rules and regulations are taught. (And yet, crime of crimes, geometry is gone! In Sweden and perhaps elsewhere, high school students do not learn—see below—any demonstration of the Pythagorean theorem.) When entering the realm of stochastics, therefore, a student comes with preconceived ideas that are hard to break. So we end up teaching in a way that leaves the interesting math for later (or for never). Stochastics needs, at a fundamental level, intuition that is distinct from “Newtonian math”. And one has to work hard if one is to pass the message properly to the uninitiated. For example, when we want to argue that ℙ(A) is less than or equal to ℙ(B) then we really are working at the logic level: A implies B. This innocuous thing, something that we may smile at, is not something we teach at the Newtonian level.
As for measure theory, why not have it as part of a beginning course, just like we have some elements of analysis as part of a calculus course? I’m not suggesting proving Carathéodory’s theorem, but merely present a way for a student to become familiar with the language, some tools, and usage of the subject. Too controversial? Perhaps, but it can be done if we steal some time from endless calculus drills. Because stochastics is more fundamental to the whole of mathematics than ever before.
Learning:
I must insist that nothing can be learned unless understood, at some level. If a proof is possible at the student’s level, one must learn it (or them). For more advanced topics, there is always something that we can explain, even when we teach “stats for poets”. Say a student tells you that a normal density is a bell-shaped curve and you wish to convince him or her that that’s not good enough. Well, do a scatter plot of 5000 points (x, y) drawn from the product normal density. You see a fuzzy circle:
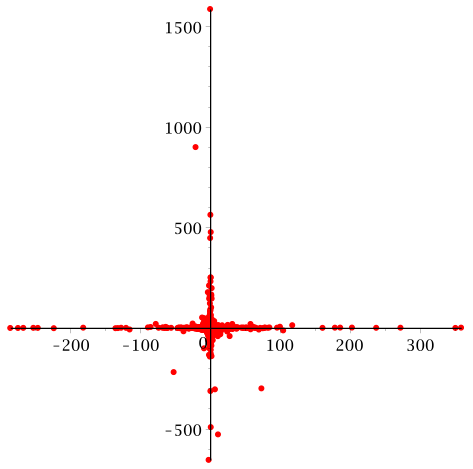
Repeat with, say, a Cauchy density which, to me, looks like a bell-shaped curve too. You get a fuzzy cross:
At this point, sub-exponentiality can also be invoked or explained: if x+y is big, then chances are that either x or y (but not both) is big. This should trigger the students’ curiosity, and the better ones will ask questions. The point is always to trigger curiosity and to provide constructive challenges.
Proofs:
Do you do proofs, or not? For me, it depends on the level, background, and goal. For example, schoolchildren must learn a proof of the Pythagorean theorem in order to learn it. I insist that every undergraduate probability course have a proof of the strong law of large numbers for an easy case (Bernoulli variables). That’s instructive and not hard at all. (After all, we are talking about the fundamental theorem of probability.) A proof is not just a means of establishing the veracity of a statement. Each proof tells its own story and gives new light. Algebra colleagues do proofs in their courses right away. A student ought to know not only the formula for the geometric sum p1 + p2 +…+ pn, but also why it is so. Here is a personal story again: A student once thought he had to compute 1p + 2p +…+ np instead of the above sum. “That’s harder,” I said, “but, if p is a positive integer then it’s also a geometric sum, if you view it the right way”. Well, the student turned out to be a bright one so I could show him what the “right way” was. (Question to the reader: do you see what I mean? And do you see why this sum is relevant to probability?)
At a more advanced level, I advocate rigor but also perspective. In particular, give a perspective of why stochastics is important both in applications and in other fields of “deterministic” mathematics. Persi Diaconis told me once that a famous analyst had asked him what’s so special with a probability measure. This sort of thing can be discussed in a more advanced class, as a starting point for giving connections. The usual remark that probability is just measure theory together with independence is as misleading as saying that Erdős’s probabilistic method is just the first axiom of probability theory (the empty set always has probability 0 or, equivalently, if there exists a ℙ such that ℙ(A) > 0 then A is nonempty). Because this does not convey the struggle, for example, of Yitang Zhang (and of Daniel Goldston, János Pintz and Cem Yıldırım) who showed, essentially using this method, that there is a c such that there are infinitely many successive primes at a distance less than c (which is probably 2). Probability measures come with a whole bag of intuition, new ideas, new techniques, new problems and new ways of thinking. Who would have thought that coupling can be useful in analysis? For example, (regular) conditional probability provides a means for constructing a natural measure on a manifold by “restricting” the natural uniform measure sitting in the ambient space through a procedure called disintegration (see, e.g., J.T. Chang and D. Pollard, Conditioning as disintegration, Statistica Neerlandica 51, 287–317, 1997). The statistical way of thinking offers a lot to many fields of mathematics. At the minimum, it often guides one to ask the right questions. The list is very big but I’m constrained here by space.
In closing, I am reflecting on Yoram Gat’s column in the October/November 2017 issue, “Learning as the replication of knowledge” in which he rightly wonders, inter alia, if there are lessons devoted to how learning occurs. In several countries in Europe, younger faculty members are supposed to be trained by attending very lengthy pedagogical courses. However, I have not met any single individual (in mathematical sciences) who has found these courses useful at all, other than learning some practical matters. Those courses advocate the point of view that learning is independent of the subject or the discipline. I do not agree with this. In my opinion, and experience, learning mathematics is very different from learning literature, although I would be the first to recommend to a mathematician to spend some time reading good literature.
A question
A question to all of you, then: what is your opinion and experience of pedagogical courses? In my accumulated experience, I have observed that independent thought is increasingly discouraged. And that the system of rewards (exams) is very little correlated with knowledge obtained. In a large number of universities, students receive vocational training, they learn by rote and are merely being taught how to pass exams. Last but not least, let us take a look at how students are prepared in schools. In the Swedish educational system, mathematics has virtually been eliminated from schools. Some more daring schools offer courses in gaming, while others make it mandatory that students take a class in playing Minecraft as they think that this encourages thinking. Does it?

Does teaching Minecraft in schools encourage children to develop their thinking skills? Photo: Wikimedia/Kevin Jarrett


1 comment on “Takis Tackles: Mathematical Education”